"What's time got to do, got to do with it.
What's time but a second hand in motion."
(with apologies to Tina Turner)

The first consequence of the two postulates is that distant events which are simultaneous in one frame may not be simultaneous in another frame.

The first step to establishing this result is to have an operational definition of "simultaneous."

OPERATIONAL DEFINITION OF "SIMULTANEITY OF SEPARATED EVENTS:"

Given two events, they are simultaneous if an observer placed in the middle of the line in space joining the two events receives light signals of the events at the same time.

The observer knows he is in the middle of the events by placing meter sticks end to end and noticing that it takes as many meter sticks to reach from the observer to event 1 as it does to reach from the observer to event 2.

Note that this definition would not be so good if c were not constant.

If, on a windy day, an observer was placed midway between two firecrackers and heard them at the same time she might not conclude that they went off simultaneously. Since the sound travels relative to the air (its "ether") a wind blowing from one firecracker towards the other will result in sound from one firecracker being "wind aided" while the sound from the other firecracker will be "fighting" the wind. The observer could then measure the speed of the two sound signals and, noticing the difference in speed decide that the one which had slower sound speed must have popped first. Its sound had to travel the same distance as the faster-sound signal but since its sound traveled slower it must have popped first. To use the new operational definition with sound (which is not the same speed for all observers..at least when the wind blows) would throw out our previous understanding of simultaneity.

The definition with light signals suffers from no such flaw since by postulate 2) both signals travel the same distance at the same speed, c. Thus if they arrive together they must have started at the same time. This definition is in accordance with any and all previous intuitive but non-operational definitions. Nothing has been lost.

Now we present a Star Wars version of Einstein's original argument for which he used trains.
 We will use space trains.

PRESENTING THE SPACE TRAIN:

The space train is one light minute long. The pilot's cabin is in the center of the space train and is represented by the dot in the middle. The pilot has closed circuit cameras in his cabin looking down each "arm" of the space train so he can see both ends on his split-screen monitor.

The Scene

In a section of space far from any galaxies a space train is floating peacefully at rest. Another space train zips by at a tremendous speed. A few days later (what's a day there??) the two pilots meet at a bar. The first pilot says, "Boy, you were really going fast when you passed me a few days ago."
"What do you mean?" says the second pilot, "I was standing still and you zipped past ME like a bat out of Andromeda."

Who is right? Well, both and neither. Each is looking at things from their own inertial frame. Each one considers them self at rest and the other moving relative to them. According to postulate 1) they are each as right as the other.

The Accident as Observed by A:

Now we throw some events in.

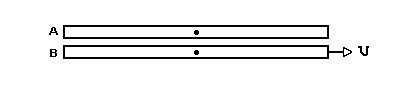
This is a schematic diagram of what happened according to A's point
of view. A is the pilot in the space train marked "A." According to
the pilot of A her space train was not moving. Space train B came zipping
by at speed c/2. Suddenly a meteor hit the left ends of the two trains.
AT THE SAME TIME (we'll get to that) another meteor clips off the right
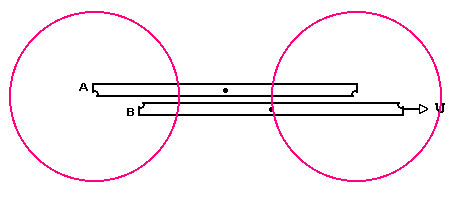
ends of the two trains. At that instant it looked like this:

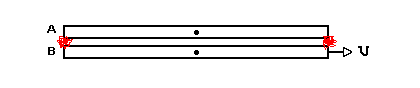
Now neither pilot knows what has happened yet since the light signal
has not reached their cabin. A bit later the situation looks like
this (where the red circles indicate the expanding edge of the light
signals from the two explosions)

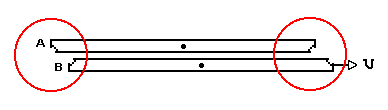
Still neither pilot is aware of the hits. A bit later the picture
looks like this:

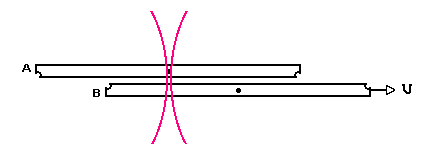
At this point in time (Thank you Watergate) the pilot of train B
has received the light signal. "Oh no!" she says, "My front end
has been hit by a meteor.

The pilot of A on the other hand thinks everything is fine. In this
frame B has seen the front hit first because she is traveling toward
the event and thus encounters the signal sooner than A.

A bit later:

Now A says, "Wow, simultaneous hits at both ends."
B says, "At least my left end is OK."

A bit later:

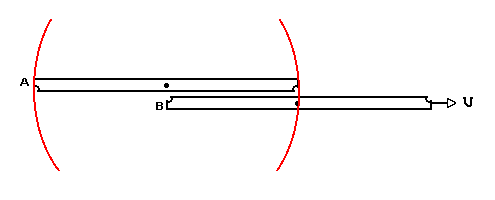
Now the pilot of B sees the left hit. "Oh no! Now my other end has been hit."

The question is: "were the hits simultaneous?"

Well, In A's frame of reference they were. A is in the middle of her ship and the light signals reached A at the same time. The operational definition for simultaneity is satisfied. However, in B's frame of reference they are not simultaneous. B is in the middle of her ship but saw the right hit well before the left hit.

"But," one may protest, "B moved away from the left event and toward the right event. She wasn't in the middle of the events when she observed them." But in B's point of view it is A's space train that is moving and in B's frame B is not moving. The "points is space" where the hits occurred stay at the ends of the their own space trains in each pilot's inertial frame. After all, each pilot considers herself at rest. In each pilot's frame it is the other train that is moving away or toward the "points in space" where the events happened.

Also, each pilot can measure the speed of the light signals through the cabin. They will by postulate 2) get good old c for a result. Since the signals traveled at the same speed and, as measured in each frame, traveled half the distance of the train they each took the same time in transit. Thus in A's frame the hits were simultaneous but in B's frame they weren't. In B's frame the right hit happened first and the left happened later.

The interesting thing is that both A and B are "right." There is no way to objectively decide that one's interpretation of the events are correct and the other's incorrect.

This leads to our first "weird" result of Special Relativity:
 

RULE 1. Separated events which are simultaneous in one frame
are not necessarily simultaneous in another.

In fact if there were a third space train, C, traveling to the left at c/2 (as measured in A's frame) It would conclude that the left hit occurred before the right hit.

So:

• In A's frame: The hits are simultaneous.
• In B's frame: The right hit happened first.
• In C's frame: The left hit happened first.

and they all are correct.

Notice that the following events will be in each pilot's log:
 

• Meteor hits left end.
• Meteor hits right end.
• B sees right hit.
• A sees both hits simultaneously.
• B sees left hit.

There is no question between the pilots that these events happened. The only question is the interpretation of them. It is this interpretation that is relative.

THOUGHT QUESTIONS: How is the concept of time needed when measuring length? What does all the meteor-hit stuff look like in B's frame?

These will be discussed in:

 Next: 4. How Do You Measure a Fish?

Send any comments, criticisms or reactions to:

 dogschool@dogmail.com